Complex Number
Integrator & Differentiator
The way we installed Ubuntu
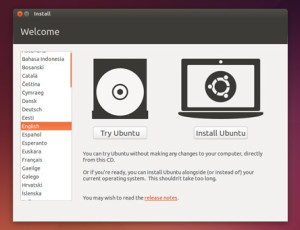
2. Prepare to install Ubuntu
We recommend you plug your computer into a power source
You should also make sure you have enough space on your computer to install Ubuntu
We advise you to select Download updates while installing and Install this third-party software now
You should also stay connected to the internet so you can get the latest updates while you install Ubuntu
If you’re not connected to the internet, we’ll help you set up wireless at the next step.
3. Setting up updates
we used the APTonCD and Synaptic Package Manager application to install all the updates from another system, which helped us minimize downloading time for updates. Hence we installed Ubuntu by un ticking the box marked “Download updates while installing.”
4. Allocate drive space
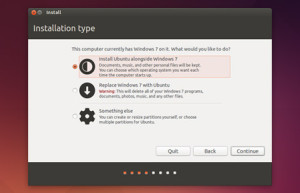 Use the check boxes to choose whether you’d like to Install Ubuntu alongside another operating system, delete your existing operating system and replace it with Ubuntu, or — if you’re an advanced user — choose the ’Something else’ option
Use the check boxes to choose whether you’d like to Install Ubuntu alongside another operating system, delete your existing operating system and replace it with Ubuntu, or — if you’re an advanced user — choose the ’Something else’ option
5.Begin the installation
Depending on your previous selections, you can now verify that you have chosen the way in which you would like to install Ubuntu. The installation process will begin when you click the Install Now button. Ubuntu needs about 4.5 GB to install, so add a few extra GB to allow for your files.
6. Select your location
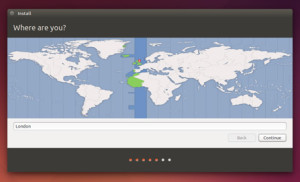 If you are connected to the internet, this should be done automatically. Check your location is correct and click ’Forward’ to proceed. If you’re unsure of your time zone, type the name of the town you’re in or click on the map and we’ll help you find it.
If you are connected to the internet, this should be done automatically. Check your location is correct and click ’Forward’ to proceed. If you’re unsure of your time zone, type the name of the town you’re in or click on the map and we’ll help you find it.
TIP: If you’re having problems connecting to the Internet, use the menu in the top-right-hand corner to select a network.
7. Select your preferred keyboard layout
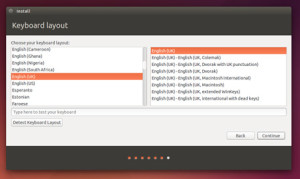 Click on the language option you need. If you’re not sure, click the ’Detect Keyboard Layout’ button for help.
Click on the language option you need. If you’re not sure, click the ’Detect Keyboard Layout’ button for help.
8. Enter your login and password details, and wait for installation
9. That’s it
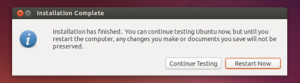 All that’s left is to restart your computer and start Ubuntuing.
All that’s left is to restart your computer and start Ubuntuing.
Comparison between Frequecy, Wavelength & Time period
FREQUENCY, WAVELENGTH & TIME PERIOD
In general, frequency, wavelength & time period are quite confusing parameters. Indeed, these three are entirely different in nature.
FREQUENCY
Frequency is the number of occurrences of a repeating event per unit time. Unit of frequency is hertz(hz).
WAVELENGTH
Wavelength is the distance between identical points in the adjacent cycles of a waveform signal propagated in space or along a wire. Unit of wavelength is meter(m).
TIME PERIOD
The time taken to complete one full cycle is said to be time period. Unit of time period is meter/second (ms).
EXAMPLE
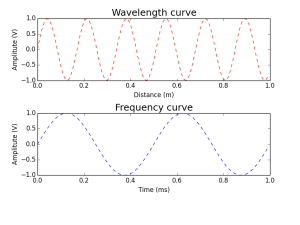
Lets take a signal at 2 kHz. Then the period is 0.5 ms. To plot this as a function of time.If a sound wave is travelling in space with a speed of 340 m/s then the wavelength is the distance the sound will travel in a period 340 * 0.5m = 17 cm. (i.e) 0.17m
PYTHON CODE
import numpy as n
import matplotlib.pyplot as p
f = 2
y = .17
d =n.arange(0,1,0.01)
t=n.arange(0,1,0.01)
wavelength =n.sin(2*n.pi/y*d)
frequency =n.sin(2*n.pi*f*t)
p.subplot(211)
p.plot(t, wavelength,’r–‘)
p.xlabel(‘Distance (m)’)
p.ylabel(‘Amplitute (V)’)
p.title(‘Wavelength curve’, fontsize=18)
p.subplot(212)
p.plot(d,frequency,’b–‘)
p.xlabel(‘Time (ms)’)
p.ylabel(‘Amplitute (V)’)
p.title(‘Frequency curve’, fontsize=18)
p.show(block=False)
WAVEFORM
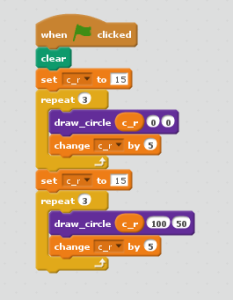
Cartesian Coordinate System
Scratch cartesian circle_s pdf
To draw a circle using Cartesian coordinate system
1.
2.
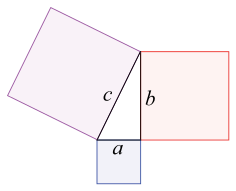
A Cartesian coordinate system is a coordinate system that specifies each point uniquely in a plane by a pair of numerical coordinates. Considering two points x and y on the x-axis and y-axis which meet at (x,y), this produces a right angle triangle with base of length x and height y. Here we could implement Pythagorean theorem which states ; The sum of the areas of the two squares on the legs (a and b) equals the area of the square on the hypotenuse (c). now applying to the above fig we get.
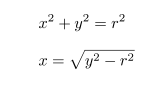 where ‘r’ denotes the radius of the circle, which can be drawn by keeping either x or y a constant and varying the others value.
where ‘r’ denotes the radius of the circle, which can be drawn by keeping either x or y a constant and varying the others value.
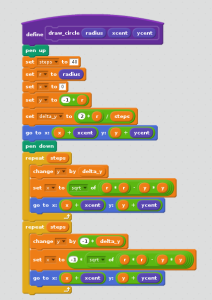
To run the script ; Script ;
- the step are defined to 40.
- setting radius of the circle to r
- defining x position to 0
- defining y position to -r ( i.e.. To start the circle from the point (0,-r))
- determines the centre of x and y.Pen down starts to draw
- the first loop repeats for the number of steps i.e..40
- y value is changed with respect to delta of y .
- x is set to the modified equation of circle i.e.. x= √(r*r) – (y*y)
- this draws only a semi circle starting from the point (0,-r) to the point (0,r)
- hence we require a second loop to draw the other half of the semi circle.
- the second loop repeats for the number of steps ie.40
- y value is changed with respect to delta of – y.
- y is set to the modified equation of circle i.e.. x= – √ (r*r) – (y*y)
- this draws only a semi circle starting from the point (0,r) to the point (0,-r)
- hence the other half of the semi circle is dawn.
Now this whole script is user defined to perform 3 concentric circles around each points (0,0) and (100,50)
The Drawn Circles;
reference : http://en.wikipedia.org – image 2
A vibrant opening
We had a fun opening with the children of Isai Ambalam School putting up a mini fair with blindfold bursting of balloons, pick out the right items (and components), dropping the coin in a bucket of water to get to target in the center of the bucket, bun eater and a recent addition the finch robot game. One of the students was also displaying the scratch programs they had written for fun and to learn various mathematical concepts.
 |
 |
 |
 |
 |
A short speech from Sanjeev was followed by the demonstration of SpeeDe our first project that detects the instantaneous speed (actually speed averaged over 6 cm) of an objects that falls through it. The project can help children understand the speed of objects dropping to the ground, the speed of a punch/kick, etc. The arduino based project used a couple of modified laser pointers and print the speed in m/s & km/hr on a 16×2 display mounted on a frame. More about the project and step by step construction will be available soon under projects.
 |
 |
This followed lighting floating candles in a large bronze vessel with water. The kolam around the vessel made this an interesting affair as well and children and adults put in their candles into the water.
 |
 |
 |
 |
We wrapped up with samosas (from Ganesh Shree in Pondy), cookies, fruits and tea. The children who had diligently kept their stalls till then dived into the snacks as well. Back at school they were raving about the samosas. There is a little project they have undertaken to draw a pie chart for the samosas that they all ate using Scratch.
 |
 |
 |
 |
Inauguration
The adventure of Aura Auro Design begins on 2 Jan 2015 @ 10:30 a.m. at Saracon Campus in Auroville.
You are welcome to attend, but please RSVP sanjeev.r@auroville.org.in to help us make arrangements.
There is a small lamp lighting, followed with games with Isai Ambalam school children and samosas from Ganesh Shree in Pondicherry. You may also stay to play some board games. Once all visitors leave we start our work.
Fraction addition
Children demonstrating their understanding of fraction addition and the common mistakes they made when they kept forgetting the procedural step of taking LCM when in 5th grade they used to just add the numerators (the denominators were the same then).
Transforming English stories into scratch
A few of the many stories that were translated into scratch by the children:
A simple project with some basic work that most children are able to do